Podcast 129 - NoBull Gas Laws (Part Two!)
- Sam Ireland
- Jul 29, 2021
- 8 min read
Welcome to round two! We'll be going over fewer laws than last time, but this will round things out nicely! We'll be covering the laws of Fick, Graham, and Dalton/Amagat. Part One of this blog can be found here. Let's get started!
Fick's Law

Fick rhymes with thick, which is the way I always remember this law. Fick's law is all about how a molecule travels from one place to another - it's all about diffusion. Diffusion is something we care very much about since we're constantly dealing with issues that involve trying to get oxygen into patients, and carbon dioxide out (oxygenation and ventilation). There are a few simple parts to this law, and a few not so simple. The graph above involves the concentration of a molecule and the thickness of a membrane. That makes sense because we would naturally hypothesize that a very thick membrane with a low concentration gradient would result in a very slow rate of diffusion. Alternatively, a very thin membrane with a high concentration gradient would result in a very fast rate of diffusion. We can add one more thing to this equation as well, which is the weight of the molecules. Molecules like helium (found in gas mixes such as heliox mentioned down below in Graham's law) will diffuse faster because they're smaller and lighter.
However, let's assume that we have a set molecule size for the rest of this discussion on Fick's law, and assume we're using all oxygen molecules (100% oxygen / 1.0 FiO2). That leaves our other factors for us to attempt to manipulate. For this thought experiment, assume you could directly alter the area around the alveoli and the capillary around it. What would you do to increase the diffusion of oxygen to the maximum possible amount? Take a look at this next picture and think about how you would alter the factors given (keep in mind the rate of diffusion is a product of the other factors).

If we can't directly influence the rate of diffusion, and we're stuck with one molecule size, what's left? Well, we could do a few things.
Increase the concentration gradient
Decrease the thickness of the membrane
Increase the surface area
Maybe that would end up looking something like this next picture.

Increasing the concentration gradient is pretty simple - we just turn up the percent of oxygen by giving either a higher flow of oxygen (to take up more of their inspiratory flow demand), directly alter the percent of oxygen (turn up the FiO2), or both. What about the surface area?
The surface area can be an increase on the alveoli side, the capillary side, or both. On the side of the alveoli, we could apply pressure to distent the alveoli. On the capillary side, perhaps we could apply a pulmonary artery vasodilator such as epoprostenol, inhaled nitric oxide, or nebulized nitroglycerine. Is there anything we can do about the thickness of the membrane?
Yes, but sometimes it's harder to do this than others. It also depends on what you're qualifying as a membrane. If you consider this semi-permeable membrane to include things that induce pulmonary shunt, there is a lot we could do about that. Pulmonary edema comes to mind as an easy example, in which PEEP would assist in overcoming the surface tension of the water and thin this 'layer'. However, we can also have issues between the alveoli and capillary themselves, such as interstitial edema in the lungs - this will be more difficult to get rid of. This doesn't just include edema but would be any kind of interstitial lung disease. A respiratory therapist or pulmonologist can actually measure the health of this membrane by running something called a DLCO test.
Here is the approximate graph for Fick's Law. Sometimes it helps to animate when there is an extra axis:
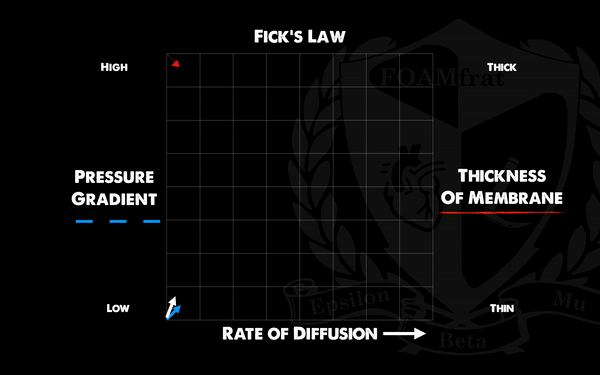
Image version. Also, when you're looking at a graph like this, sometimes it's easier to compare the X-axis to one side at a time. Compare X to Y, and then X to Z (I'm calling the second Y-axis a Z). Comparing Y to Z doesn't give you much information without realizing the rate of diffusion (X).

Graham's Law

For all the gas laws that we've talked about, we've really only hinted at diffusion and effusion. Graham's law is the law of diffusion and effusion, and when we talked about molecular weight up above in Fick's law, we borrowed a lot of Graham's law. Before we talk about clinical application, let's quickly clear up what the difference is between diffusion and effusion (even though they're used interchangeably in this law due to the same action density has on them).
Diffusion is the spread of a molecule in the open air (or liquid). If you light a candle in the corner of a room, will the smell stay there? no, it will spread throughout the room. Why? Perhaps the easiest way to illustrate diffusion is with this classic box example.

Here we see box number 1 has a combination of oxygen and carbon dioxide, and box 2 has pure oxygen. What will happen to the mixtures of gas if we remove the partition in between the two boxes? Will the CO2 stay where it is, or not? Let's see!

Notice what happened? The CO2 went down its concentration gradient until it was evenly dispersed throughout the box. Why did this happen? As molecules float around, they are constantly colliding with each other. This has to do with the kinetic molecular theory. Essentially what is happening is that as molecules collide, they all get mixed up. Smaller (lighter) molecules move faster, and larger (heavier) molecules move slower. This gives the lighter molecules a higher chance of spreading farther in less time. This theory of particles moving down their concentration gradient works in liquid as well. I saw a news article that fits the example perfectly. Behold! Diffusion... Demystified!

Would you want to go in the water anywhere around that mess? No. Why? Because particles move down their concentration gradients. I suppose a less gross example would be dropping some dye into clear water. Eventually, the dye will disburse evenly. What about effusion?

Effusion is different from diffusion because there is not an unlimited amount of space for the molecules to move around in. In diffusion, molecules freely collided and could go wherever they wanted. In effusion, there is a smaller opening for the molecules to get through. Graham's law speaks about the time of effusion of one gas versus another. As you can see above, the small, lighter molecules are winning the race through the opening. This is because they bounce off of other molecules more quickly and more frequently, so they have increased chances of bouncing through the hole.
When it comes to oxygen, there really isn't anything we can do about changing that molecule, but what about the rest of the air? We could remove nitrogen and swap it out with helium (a very light gas), and that would increase the rate of diffusion from one area to another. This strategy is commonly employed when heliox is used for asthma patients (and others with issues getting past an obstruction). Heliox can be in a mixture of 80:20, 70:30, and 60:40 helium to oxygen ratio. You actually have to do a calculation for your flow meter because heliox flows so much more quickly from the bottle to the patient. For example, if you're using 80:20 heliox, your flow coming out of the regulator is actually 1.8 times whatever you have it set to (so a reading of 10 LPM would actually be a flow of 18 LPM!).


This is the same reason why heliox works so well in asthma. The lighter particles (helium as opposed to nitrogen or oxygen) bounce faster and more frequently, allowing them to get through tighter spaces more quickly. However, this has clinical application is more than just heliox therapy.

See the similarities to the two-box illustration? Except, apneic oxygenation has some other advantages, in that it doesn't just have to rely on passive, slow effusion. High flow oxygen via nasal cannula keeps the turbulence high. I'm not sure if you've ever tried to turn up a nasal cannula flow on yourself (up to 15 or 30+ LPM), but it feels like a jet stream on your glottic opening. When we're providing that much particle collision on one side of the glottic opening, it's no wonder that apneic oxygenation can keep saturations so high for so long (especially when the body is actively absorbing oxygen from the alveoli at a rate of ~250mL/min even during apnea).
And by the way... We've known about apneic oxygenation since before the 1950s. This is a snapshot from a study done in 1958 in which they had 8 surgical patients on apneic oxygenation for between 18 and 55 minutes. Everyone woke up fine, and not one subject desaturated below 98% SPO2. This study is linked in the very last reference down at the bottom of the page. Incredibly interesting! It's a must-read.


Dalton's Law and Amagat's Law

Dalton's law is the law of partial pressures. Dalton's law applies whenever there are gases combined that don't have violent reactions with each other. But what's a 'partial pressure' anyway?
A gas makes pressure because of molecule collisions. As collisions happen, the gas exerts pressure against the side of a container as its molecules slam into it. That's pretty easy to understand, but what if there are multiple different types of gas in the container, like oxygen and nitrogen? If we were to measure the total pressure that this mixture of gas is exerting (the oxygen and nitrogen together), we would come up with the total pressure of the gas mixture. However, what if you wanted to know how much each of the gasses is contributing to this total pressure? That would be known as partial pressure. It's like evaluating a team of people based on how much work they get done. Sure, the team gets done X amount of work, but of the three team members, how much is each one of them contributing to X? That is essentially what Dalton's law is trying to explain - how much is each gas contributing to the total pressure.
How does this law help us clinically? Basically, if you want to pass your FP-C or CFRN, you need to be able to answer a question about this law to practice clinically ;) Here's an example of a question you might see.
Question: At 760 Torr there is 20.95% oxygen. What is the partial pressure of oxygen?
Answer: 159.22
Rationale: 760 x 0.2095 = 159.22. All you do for these questions is change the percent into a decimal and multiply in order to get the partial pressure of that gas in a mixture. It's pretty simple as long as you know the law and formula.

I wanted to throw Amagot's law into the mix here as well because it works essentially just like Dalton's law except for volumes.

This graph is a little different because it takes into account how the gases would behave if they were isolated at the same temperature and pressure as the gases when they are combined. This is why I included the little boxes around the individual gas components.
Conclusion
In these past two blogs and podcasts, I've outlined all of the gas laws that we should know as clinicians (pretty much all of them except some related physics stuff that maybe we'll get to later). In this format, it's difficult to exemplify every instance in which understanding these laws come into play. However, if you understand even just the basics of these laws, so much about oxygenation and ventilation will start to make real sense. This builds a much better clinician as opposed to one who simply memorizes treatment plans and strategies. Everything comes back to understanding the basic laws of physiology. If we can understand how processes occur normally, any alteration to these processes (and their solutions) will come to mind much more easily!
I'll be giving a talk on these gas laws in the live classes inside the refresher on 8/18/21!
Thanks for reading and listening!
References
Chandan G, Cascella M. Gas Laws and Clinical Application. [Updated 2020 Sep 3]. In: StatPearls [Internet]. Treasure Island (FL): StatPearls Publishing; 2021 Jan-. Available from: https://www.ncbi.nlm.nih.gov/books/NBK546592/
In Clark, D. Y., In Stocking, J. C., In Johnson, J., In Treadwell, D., In Corbett, P., & Air & Surface Transport Nurses Association (U.S.). (2017). Critical care transport core curriculum.
American Academy of Orthopaedic Surgeons (AAOS), UMBC, & American College of Emergency Physicians (ACEP). (2004). Critical Care Transport. Sudbury, MA: Jones and Bartlett.
Hess DR, Fink JB, Venkataraman ST, Kim IK, Myers TR, Tano BD. The history and physics of heliox. Respir Care. 2006 Jun;51(6):608-12. PMID: 16723037.
Here's a link to an interesting paper from the 1950s about prolonged apneic oxygenation that they used to call "diffusion respiration". It's a wild read about not breathing, arterial hypertension from sympathetic panic, and sodium/potassium increases (cation) while anions increase.












Comments