Inotropy Isn't 'Like a Rubber Band'
- FOAMfrat

- May 21, 2021
- 10 min read

More stretch = more contraction, right? This is often what is said about the ventricles of the heart and their ability to contract with more force the more they're stretched. People often use the illustration of a rubber band to explain this function, but that's not always a satisfactory explanation...
Instructor: The ventricles are like a rubber band - the more you stretch it, the more forcefully it will contract.
Student: So we can just always give fluid and it will increase blood pressure?
Instructor: Well, no - because there's a limit.
Student: What do you mean?
Instructor: Like a rubber band that you stretch too far and it breaks.
Student: So the heart snaps and breaks?
Instructor: Well, again, no - but it kind of stops working as well in different stages if the volume is too high.
Student: So... it's only like a rubber band sometimes?
Instructor: Exactly!
Student: .....ok cool thanks
I've heard versions of that conversation more times than I can count. It is generally true that as you stretch the heart more, it will contract harder. However, it's a little more complicated than that. The heart doesn't all of a sudden just 'snap' and then it's broken and doesn't work anymore. Alright, so... if the heart isn't like a rubber band, what is it like?
I'm going to be using an illustration that is not mine to better show the varying relationship between stretch and contraction. This illustration has been used by many people, and it's time to start using it again, because it makes a lot more sense than the rubber band thing (although it takes a little longer to explain). This illustration is about congestive heart failure, but it's all very fluid-related and applicable to this issue of fluid responsiveness. You've probably already figured out that we're going to discuss the Frank-Starling Curve - nice! We'll also throw LaPlace's Law into the mix after that!
The legs, the hill, and the box.
This illustration is of a man trying to carry a box up a hill. His goal is to throw the box over the edge, so that the box lands on the X.
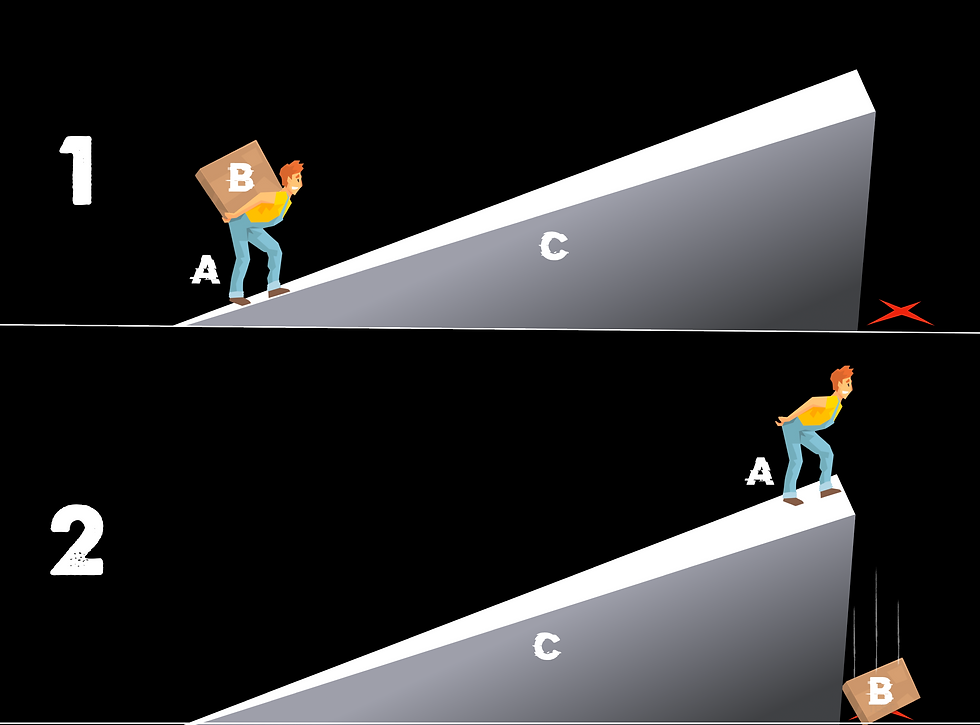
Several things must occur for this whole scenario to work out.
His legs have to be strong enough to carry the box.
The angle of the hill can't be too steep.
The load can't be too much for him to carry.
We'll start with the legs and angle of the hill, because the box is what we're trying to get at.
Chicken Legs
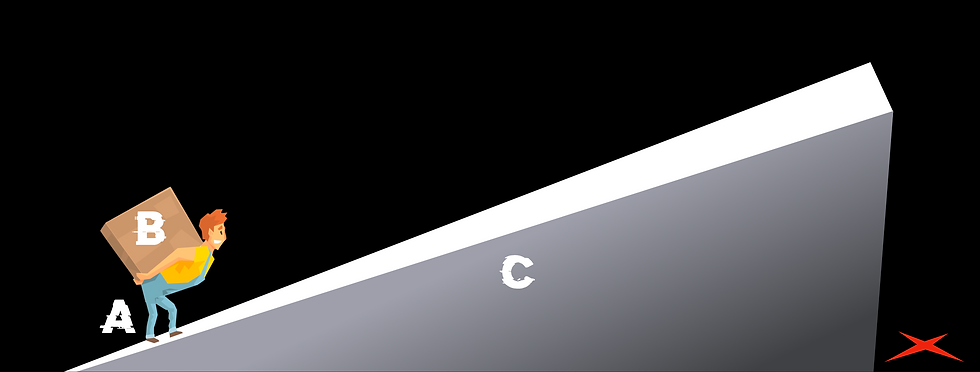
Look at those little things! This guy has no chance of getting up that hill with that box. What does this illustrate? The strength of the legs is meant to represent the strength of the ventricle. What can make the ventricles weak? Ischemia/infarction, electrolyte imbalances, cardiac-depressant drugs, and acidosis, to name the big ones.
Ninja Warrior Wall

This wall reminds me of American Ninja Warrior when the contestants have to run up the wall to get to the buzzer at the end of the course. Clearly, this guy isn't getting up that wall - it's way too steep. This is meant to represent afterload / systemic vascular resistance. If the afterload gets too high, it's really hard for even a healthy heart to pump against it (notice the legs are normal size in this picture).

Time Trials
Here's the part of the illustration I wanted to arrive at. Let's see how much this guy can carry. We're going to continue to add boxes to his back, and see how many he can delivery in a minute!
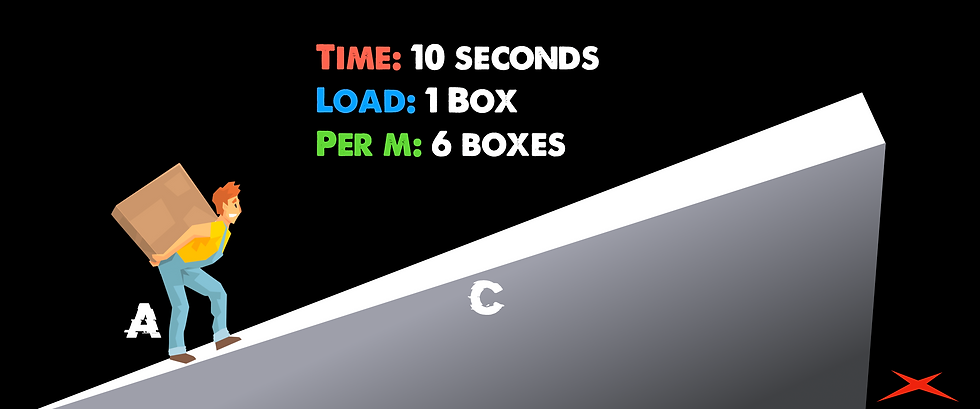
Alright, not bad. He's carrying one box, and he can make a roundtrip in 10 seconds. Sounds like he could carry way more! Let's add some volume.
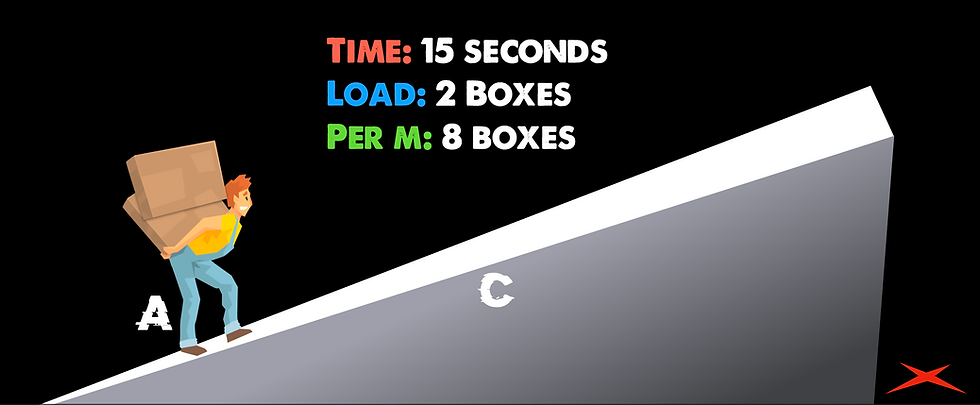
Looks like he handled it pretty well! We added a box, and he can make a roundtrip in 15 seconds. That brought his average per minute from 6 to 8! Let's see how far we can push this.
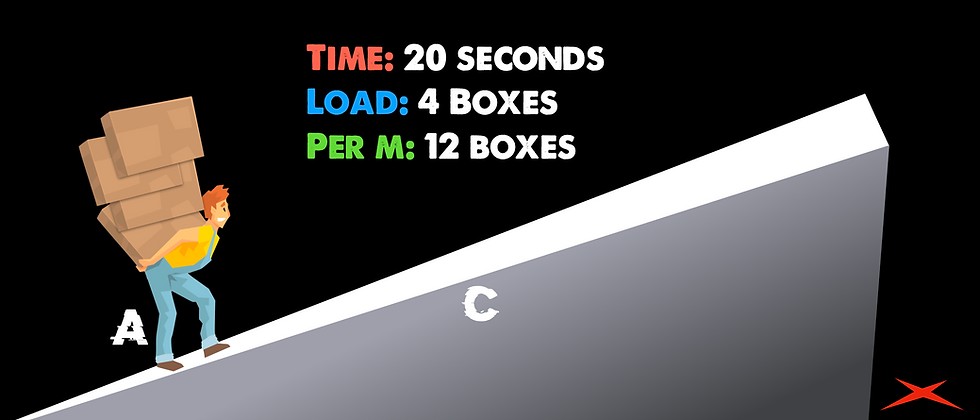
Still going! He's slowing down under the increased volume for sure. It's now taking him twice as long as it did when he only had one box, but he's still increasing his average per minute.

Hmmmm. We added a couple more boxes, but we're not seeing any increase in delivered boxes per minute as compared to last time. It's taking this guy so long to work under that increased volume that adding the extra volume didn't increase how many were delivered per minute. This is called hitting a plateau. What do you think will happen if we increase the volume even more?
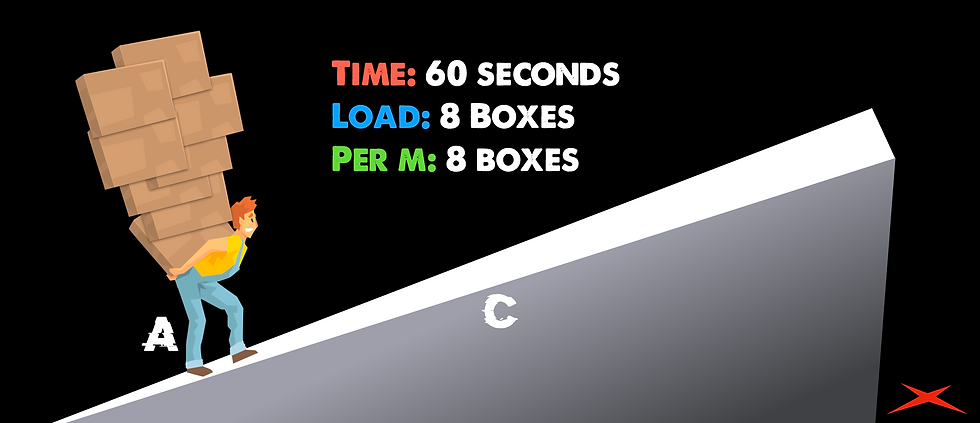
Uh oh. We've pushed this guy too far. The volume is now so high that his delivery rate per minute is actually going down - he's overloaded - the volume is too high for him to handle.
Did you notice the trend? As we increased the volume little by little, we saw a steady increase in the amount of output. However, we then hit a level of volume where output didn't increase - a plateau. Finally, we saw that a volume overload could actually hurt output. If we were to graph this out, it would look very familiar.
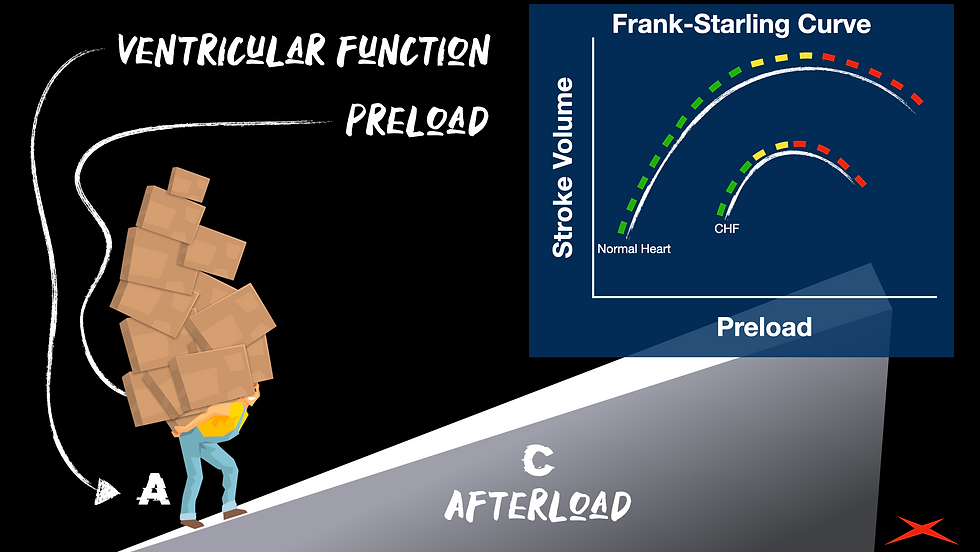
The green parts of the curve is where adding volume increased output. But, remember when we added volume and hit a plateau? That's the yellow part. Finally, we added too much volume, and the output actually decreased (that's the 'dark side' of the curve - red).
The plateau phase and the decompensation phase (yellow and red) are phases that cannot be explained with the classic 'rubber band' explanation. Sure, the green part works with the rubber band illustration, but that's where it stops. Another aspect of that graph above is that the CHF patient might not have far to go before they plateau and decline due to fluid overload. In fact, they might already be in that state of decline when we find them (usually with a bunch of afterload and a weak ventricle to add insult to injury). Don't worry - if this illustration seems like too much to remember and explain, just show someone this blog ;)
Thanks Otto and Ernest...
But don't forget about Pierre!
Otto Frank and Ernest Starling really helped us understand how the ventricle responds to volume. However, we can also look at this in a mathematical way, which is a nice cherry on top of the Frank-Starling Curve. Let's check out how Pierre-Simon Laplace's Law can apply to ventricular volume!
What's the biggest drivers of myocardial oxygen consumption? The main contributor is heart rate, but there are a few other really major players in how much oxygen the heart is using, such as inotropy, afterload, and yes - preload plays a role in all of this. Basically, however tense the wall of the ventricle has to be, the more oxygen it's going to consume. If we're dealing with a cardiac patient, we don't want the heart to be under a lot of stress (a high oxygen demand). What do you think - could we measure Myocardial wall tension if we wanted to?
I have no idea. But, what I do know is that we could find some numbers that are pretty close and play around with them. Let's look at the information we would need in order to estimate the wall tension. Oh, and these factors will also tell us why the Frank-Starling curve looks like it does - why we reach a point of diminishing returns with volume. We will need to know two things:
The peak systolic pressure (probably from the LV, but we'll substitute for SBP).
The radius of the left ventricle at the end of diastole. (Could we get this from a mid-LV parasternal short-axis view with ultrasound?)
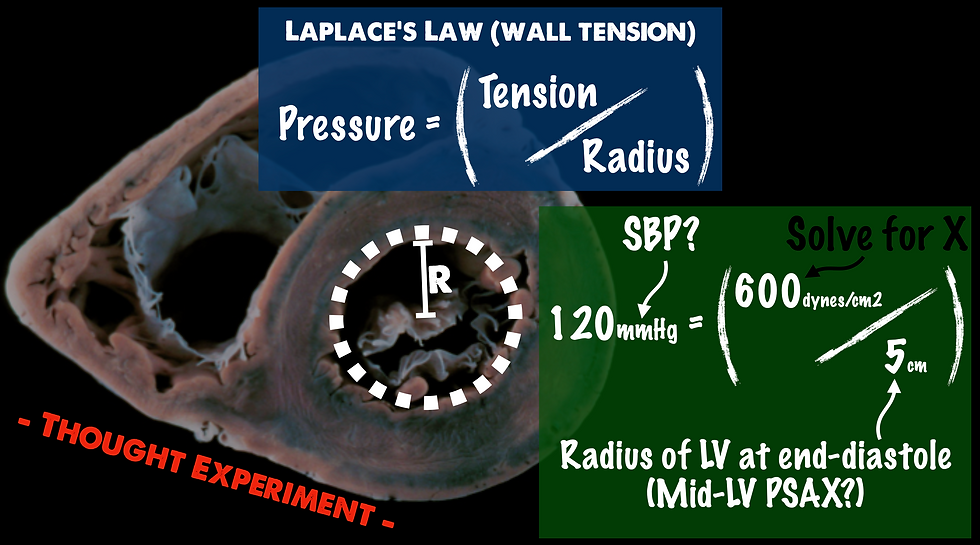
In this thought experiment, you would just take your systolic blood pressure and multiply it by the radius of your LV at the end of diastole. The result would be solving for X, which is your dynes/cm2. Now... before a physics nerd strangles me, I will admit that this math is far from perfect the way that I have it laid out here. For one, there are variables when it comes to the difference in pressure from ventricular to systemic, and valve issues can exacerbate that discrepancy. Another issue is that these equations usually assume a perfect sphere or right cylinder, but the ventricle is a rather irregular shape. I could also see an issue where wall motion abnormality could disturb how the radius is measured and not equally account for the wall tension. Aaand I could go on and on... However, the irregularities are consistent, not inconsistent. Perhaps the number obtained from doing this test would be admittedly flawed, but could be looked at as a trend. What would you use it for? I'm not sure about that either, yet - but it's pretty cool to think that we could trend this type of value with ultrasound. This is something I'll be giving more thought to. Let me know in the comments what you think of measuring this! What's the use case?
Alright, so how does Laplace's Law help us understand the math of fluid responsiveness? Let's assume that the above example (600 dynes/cm2) is the max contractile force of the ventricle - the patient's heart cannot contract any harder than they already are. So, if this number can't change, can the diameter? Yes! If volume is added (like when we give fluid), the diameter is going to increase. So, let's see how this affects the pressure produced.
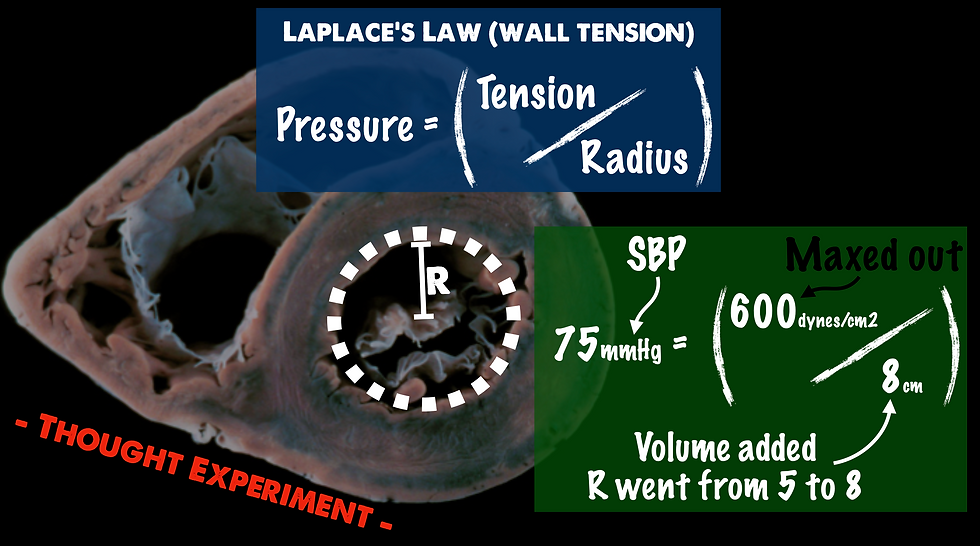
When we divide that maxed out 600 dynes/cm2 by a bigger number (because the radius went up), the result is a smaller number (our systolic blood pressure went down). This is the mathematical representation of the 'dark side' (red part) of the Frank-Starling Curve that we looked at above.
This decrease in contractile force with more stretch reminds me of lifting weights. Think of someone doing simple bicep curls. If you grab some dumbbells, you would probably start off using great form by using a full range of motion. However, as you get tired, your form starts to fail. If you needed to keep going, you would start to decrease your range in motion in order to keep pumping out repetitions. If someone forced you to extend your arm fully while it was tired (go back to full range in motion after resorting to cheater / short range of motion repetitions), you would likely not be able to break the angle of the elbow to start another repetition. To put it simply, when a muscle is stretched to the max, it gets very difficult to contract from that position. Kia Green can tell you all about it.

Conclusion
Whenever we hear about laws such as the ones considered in this post, it can initially be difficult to extrapolate where the clinical application lies. When it comes to the Frank-Starling Curve, think about where your patient might be on that curve (it helps to visualize it based on their response to volume). With LaPlace's Law, we start to realize that a maxed-out ventricle only loses contractile force once it's dilated further (which we demonstrated with our equation above). These laws might also give us an idea why it's good to give set amounts of fluid so that we can observe the patient's response to specific volumes. So is inotropy really like a rubber band?
Inotropy is like a rubber band at first, but I feel that it's much more nuanced than that. We have a tendency to over-simplify complex subjects, and this is definitely one of them. Cardiovascular physiology is one of the key aspects to emergency and critical care, and we should know the laws that govern this physiology more deeply than simple baseline knowledge and illustrations. Hopefully this blog helped you gain a deeper understanding on the squeeze of the heart and how to better illustrate it!
Peer Review by Tyler Christifulli
The question of fluid responsiveness continues to be one of the most challenging in endeavors in medicine. A functional knowledge of the Frank-Starling Curve and LaPlace's Law can help the clinician understand why specific pathologies change the starting point in which one reaches diminishing returns with increasing preload. As mentioned in the article, wall tension is second to heart rate in terms of myocardial oxygen consumption (MvO2). As ejection fraction (EF) decreases, the left ventricular end diastolic pressure increases. This means not only are we using more oxygen, but we are inhibiting delivery due to increased resistance to coronary blood flow. There are a few ways we can estimate decreased EF.
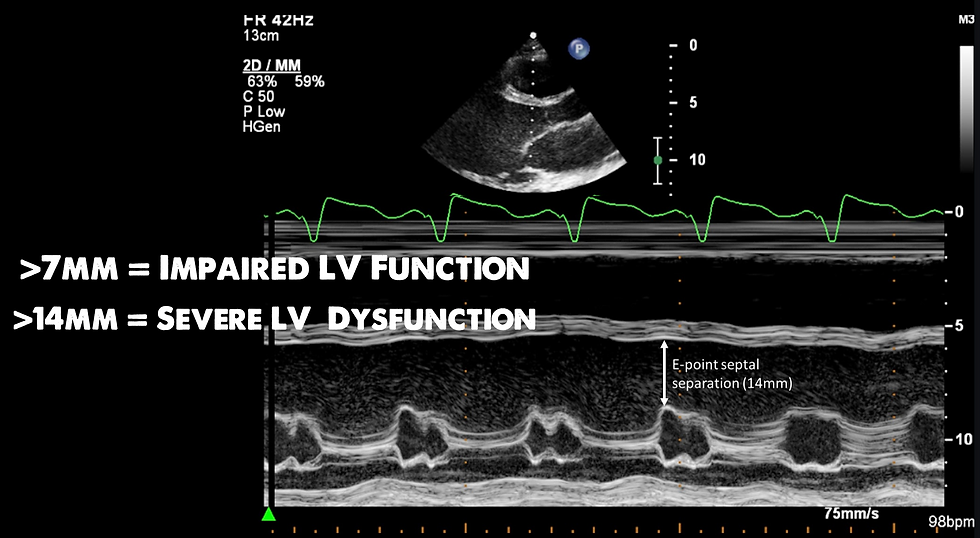
E-Point Septal Separation
The mitral valve will open twice during diastole. The first time it opens is because of a pressure gradient directly following systole. Right after the LV ejects blood, a condition is met in which the pressure in the left atrium is higher than the pressure in the LV. The degree of this pressure differentiation can be estimated by looking at the mitral valve in relation to the septum. As we get older, the heart loses the ability to relax and the tense state of the heart during diastole decreases the pressure differentiation the first time the mitral valve opens (passive filling). This may get the clinician an idea of wall tension and insight into fluid tolerance.
Velocity Time Integral
One of the components of the cardiac output formula is something called the velocity time integral (VTI). This evaluates how fast the column of blood is moving through a point of reference during systole. It is measured using a pulse wave doppler over the left ventricular outflow tract. I think of it like looking at how fast the guy is walking up the hill in Sam's earlier illustration.
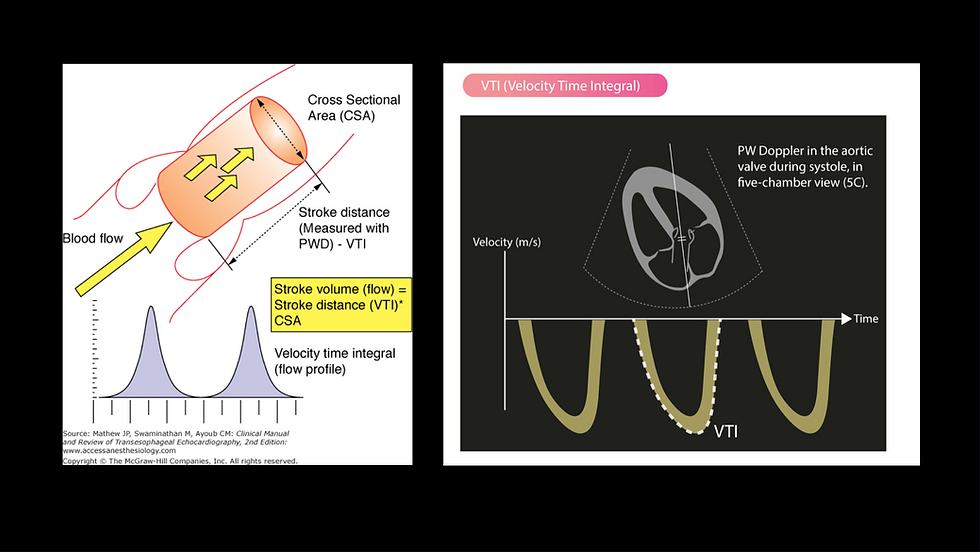
As sam mentions in his thought experiment, using the radius of a parasternal short axis is prone to inconsistencies when wall motion abnormalities are present and uniform contraction deteriorates.
If LV wall tension is the question, I believe evaluating the E wave during passive filling on ultrasound gives better insight and can be done at the bedside.
If fluid responsiveness is the question, VTI has been shown to be accurate and consistent.
Blanco, P. Rationale for using the velocity–time integral and the minute distance for assessing the stroke volume and cardiac output in point-of-care settings. Ultrasound J12, 21 (2020). https://doi.org/10.1186/s13089-020-00170-x
Now Checkout The Podcast!
References
First, a couple websites I love:
https://www.cvphysiology.com/Cardiac%20Function/CF003
https://derangedphysiology.com/main/cicm-primary-exam/required-reading/cardiovascular-system/Chapter%20027/frank-starling-mechanism
This NCBI article is pretty substantial:
Delicce AV, Makaryus AN. Physiology, Frank Starling Law. [Updated 2021 Feb 17]. In: StatPearls [Internet]. Treasure Island (FL): StatPearls Publishing; 2021 Jan-. Available from: https://www.ncbi.nlm.nih.gov/books/NBK470295/
Here are some of the specific resources from that NCBI article that I found topic specially for our proposes:
Han JC, Pham T, Taberner AJ, Loiselle DS, Tran K. Solving a century-old conundrum underlying cardiac force-length relations. Am J Physiol Heart Circ Physiol. 2019 Apr 01;316(4):H781-H793. [PubMed]
Kuhtz-Buschbeck JP, Drake-Holland A, Noble MIM, Lohff B, Schaefer J. Rediscovery of Otto Frank's contribution to science. J Mol Cell Cardiol. 2018 Jun;119:96-103. [PubMed]
Chaui-Berlinck JG, Monteiro LHA. Frank-Starling mechanism and short-term adjustment of cardiac flow. J Exp Biol. 2017 Dec 01;220(Pt 23):4391-4398.
Ochsner G, Wilhelm MJ, Amacher R, Petrou A, Cesarovic N, Staufert S, Röhrnbauer B, Maisano F, Hierold C, Meboldt M, Schmid Daners M. In Vivo Evaluation of Physiologic Control Algorithms for Left Ventricular Assist Devices Based on Left Ventricular Volume or Pressure. ASAIO J. 2017 Sep/Oct;63(5):568-577.
Ait Mou Y, Bollensdorff C, Cazorla O, Magdi Y, de Tombe PP. Exploring cardiac biophysical properties. Glob Cardiol Sci Pract. 2015;2015:10.












Comments